Mathematics JAMB CBT Practice Questions & Answers 2021 SET 1
1. .In a class of 40 students, 32 offer Mathematics, 24 offer Physics and 4 offer neither Mathematics nor Physics. How many offer both Mathematics and Physics?
- A. 4
- B. 8
- C. 16
- D. 20
Correct Answer: Option D
2.Find the values of x for which
- A. x < 8
- B. x > -6
- C. x < 4
- D. x > -3
Correct Answer: Option B
3
Find \N\
- A. 65
- B. 23
- C. 17
- D. 91
Correct Answer: Option C
4. If X, Y can take values from the set (1, 2, 3 ,4), find the probability that the product of X and Y is not greater than 6
- A.
- C.
12 - D.
38
Correct Answer: Option C
Explanation

Each multiplication of elements gives 16 results out of which the ones that are not greater than 6 are asterisked (*) and they are 8 in numbers
Pr(product of x and y NOT > 6 ) =
=
5

The pie chart shows the monthly expenditure of a public servant. The monthly expenditure on housing is twice that of school fees. How much does the worker spend on housing if his monthly income is N7200?
- A. 1000
- B. 2000
- C. 3000
- D. 4000
6. Evaluate 5
- A. 8
- A.
18 - B. 1
18 - C.
25
Correct Answer: Option B
7. A trader realises 10x - x
Naira profit from the sale of x bags of corn. How many bags will give him the maximum profit?
- A. 7
- B. 6
- C. 5
- D. 4
Correct Answer: Option C
Explanation
Profit (P) = 10x − x 2
Maximum profit can be achieved when the differential of profit with respect to number of bags(x) is 0
i.e.
= 0
= 10 - 2x = 0
10 = 2x
Then x =
= 5
Answer is C
8. If y = 23
- 101
, find y, leaving your answer in base two
- A. 1110
- B. 10111
- C. 11101
- D. 111100
Correct Answer: Option B
9

Find the value of x in the diagram
- A. 10°
- B. 28°
- C. 36°
- D. 40°
Correct Answer: Option D
Explanation
The diagram shows angles at a point, the total angle at a point is 360
x - 10 + 4x - 50 + 2x + 3x + 20 = 360
10x - 40 = 360
10x = 360 + 40
10x = 400
x =
x = 40
10. Solve for t in the equation
- A.
913 - B.
713 - C. 5
- D. 9
35
Correct Answer: Option D
Explanation
Multiply through by the LCM of 4 and 3 which is 12
12 x(
(21 - t)) = (11 x 12)
9t + 4(21 - t) = 132
9t + 84 - 4t = 132
5t + 84 = 132
5t = 132 - 84 = 48
t =
t = 9
Answer is D
11. A school girl spends
on dress. What fraction remains?
- A.
56 - B.
712 - C.
512 - D.
16
Correct Answer: Option C
12. If
- A.
b(a+1−x)a+1 - B.
a+1b(a−x+1)
- C.
a(b−x+1)b+1 - D.
ba(b−x+1)
Correct Answer: Option A
13. Calculate the total surface area of a cupboard which measures 12cm by 10cm by 8cm
- A. 1920cm
2
- B. 592cm
2 - C. 296cm
2 - D. 148cm
2
Correct Answer: Option B
Explanation
Total surface area of a cupboard is given by the equation A = 2(lb + bh + lh) L = 12, b = 10, h = 8
A = 2((12 x 10) + (10 x 8) + (12 x 8))
A = 2(120 + 80 + 96)
A = 2 x 296
A = 592cm
Answer is B
14. Convert 0.04945 to two significant figures
- A. 0.040
- B. 0.049
- C. 0.050
- D. 0.49
Correct Answer: Option C
15. The probabilities that John and James pass an examination are
- and
respectively. Find the probability of both boys failing the examination.
A.
- B.
210 - C.
920 - D.
1120
Correct Answer: Option D
Explanation
Pr(both John and James passed)
=
x
Pr(john and james failed)= 1- Pr(john and james passed)
= 1 –
=
Answer is D
16. An arc of a circle of radius 14cm subtends angle 300° at the centre. Find the perimeter of the sector formed by the arc (take π =
- A. 14.67cm
- B. 42.67cm
- C. 101.33cm
- D. 513.33cm
Correct Answer: Option C
17. Simplify
- A. 25
- A.
15
- B. 1
- C.
125
Correct Answer: Option B
18
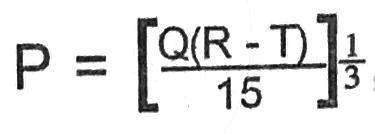
Make T the subject of the relation.
- A. T =
R+P315Q
- B. T =
R−15P3Q - C. T =R -
15P3Q - D. T =
15R+QP3
Correct Answer: Option C
19.What is the place value of 9 in the number 3.0492?
- A.
910000
- B.
91000 - C.
9100 - D.
910
Correct Answer: Option B
20. If the simple interest on a sum of money invested at 3% per annum for 2
years is N123, find the principal.
- A. N676.50
- B. N820
- C. N1,640
- D. N4,920
Correct Answer: Option C
21. A machine valued at N20,000 depreciates by 10% every year. What will be the value of the machine at the end of two years?
- A. N16,200
- B. N14,200
- C. N12,000
- D. N8,000
Correct Answer: Option A
Explanation
Since it depreciates by 10% At the end of first year, its value = 90% of 20000
=
At the end of second year, its value = 90% of 18000
=
Answer is A
22. The table shown gives the marks scored by a group of student in a test. Use the table to answer the question given.
Mark | 0 | 1 | 2 | 3 | 4 | 5 |
Frequency | 1 | 2 | 7 | 5 | 4 | 3 |
What is the median mark?
- A. 1
- B. 2
- C. 3
- D. 4
Correct Answer: Option C
Explanation
Total frequency = 1 + 2 + 7 + 5 + 4 + 3 = 22
Median is the middle number
= Nth2
term = 22th2 = 11th term
Going in ascending order, 11th term is 3, going in descending order 11th term is 3
Median = 3 + 32 = 62 = 3
Answer is C
23. The table shown gives the marks scored by a group of student in a test. Use the table to answer the question given.
Mark | 0 | 1 | 2 | 3 | 4 | 5 |
Frequency | 1 | 2 | 7 | 5 | 4 | 3 |
What is the probability of selecting a student from the group that scored 2 or 3
- A.
111
- B.
522 - C.
722 - D.
611
Correct Answer: Option D
24 A boy walks 800m in 20 minutes. Calculate his average speed in Km/H
- A. 2.4
- B. 4
- C. 24
Correct Answer: Option A
25.A car uses one litre of petrol for every 14km. If one litre of petrol cost N63.00, how far can the car go with N900.00 worth of petrol?
- A. 420Km
- B. 405Km
- C. 210Km
- D. 200Km
Correct Answer: Option D
Explanation
since 1litre cost ₦63
So ₦900 is the cost of
litres
= 14.2857litres of petrol
nbsp; The car uses 1 litre for 14km
So 14.2857 litres will be used for (14.2857 x 14)
= 200km
Answer is D
26

In the diagram, GI is a tangent to the circle at H. If EF||GI, calculate the size of ∠EHF
- A. 126°
- B. 72°
- C. 54°
- D. 28°
Correct Answer: Option B
27. How many times, correct to the nearest whole number, will a man run round a circular track of diameter 100m to cover a distance of 1000m?
- A. 3
- B. 4
- C. 5
- D. 6
Correct Answer: Option B
Explanation
In terms of distance, a circle has a total distance or perimeter of 2π or π
Where r is radius and D is the diameter
So perimeter =
x 100
= 314.2857m
To cover a distance of 1000m, he is going to round the circular track for
= 3.18
It means it is on the number 4 round after the third round he is going to cover up to 1000m
28

Use the cummulative frequency curve to answer the question. Estimate the median of the date represented on the graph
- A. 35.5
- B. 36.5
- C. 37.5
- D. 38.5
Correct Answer: Option A
29

Use the cummulative frequency curve to answer the question. What is the 80th percentile?
- A. 45.5
- B. 46.5
- C. 47.5
- D. 48.5
Correct Answer: Option C
30.
- A. 8.8 x 10
−1 - B. 8.8 x 10
−2 - C. 8.8 x 10
−3 - D. 8.8 x 10
3
31. Two sisters, Taiwo and Kehinde, own a store. The ratio of Taiwo's share to Kehinde's is 11:9. Later Kehinde sells
of her share to Taiwo for N720.00. Find the value of the store
- A. N1,080.00
- B. N2,400.00
- C. N3,000.00
- D. N3,600.00
Correct Answer: Option B
32. A room is 12m long, 9m wide and 8m high. Find the cosine of the angle which a diagonal of the room makes with the floor of the room
- A.
1517
- B.
917 - C.
815 - D.
1217
Correct Answer: Option A
33. Divide the L.C.M of 48, 64 and 80 by their H.C.F
- A. 20
- B. 30
- C. 48
- D. 60
Correct Answer: Option D
34. Find the equation of the line through (5,7) parallel to the line 7x + 5y = 12
- A. 5x + 7y = 20
- B. 7x + 5y = 70
- C. xy = 7
- D. 15x + 17y = 90
Correct Answer: Option B
35. A man's initial salary is N540.00 a month and increases after each period of six months by N36.00 a month. Find his salary in the eight month of the third year.
- A. N828.00
- B. N756.00
- C. N720.00
- D. N684.00
Correct Answer: Option C
Explanation
Since the salary increases by 36 after every 6 months
Every 6 months that can be counted on the eight month of the third year is 5
(i.e. 2 times in the first year, 2 times in the second year and once in the third year)
His salary then = initial salary + increment
= 540 + 5(36)
= 540 + 180
= ₦720.00
It can also be solves using a sequence in form of an AP
Answer is C
36. A man stands on a tree 150cm high and sees a boat at an angle of depression of 74°. Find the distance of the boat from the base of the tree.
- A. 52cm
- B. 43cm
- C. 40cm
- D. 15cm
Correct Answer: Option B
Explanation
Tan 74 = 150/x
x = 150/tan 74
= 43.01cm
37. Integrate the expression 6x
- A. 3x
3 - 2x2 + x + c - B. 2x
3 - x2 + x + c - C. 2x
3 – 3x2 + c - D. x
3 + x2 – x + c
Correct Answer: Option B
38. In how many ways can the letters LEADER be arranged?
- A. 72
- B. 144
- C. 360
- D. 720
Correct Answer: Option C
Explanation
The word LEADER has 1L 2E 1A 1D and 1R making total of 6!
=
= 360
39.
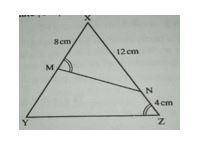
In the figure below, /MX/ = 8cm, /XN/ = 12cm, /NZ/ = 4cm and ∠ XMN = ∠ XZY. Calculate /YM/
- A. 32cm
- B. 24 cm
- C. 16 cm
- D. 12 cm
Correct Answer: Option C
Explanation
From the figure,
∠ XMN = ∠ XZY
Angle X is common
So, ∠ XNM = ∠ XYZ
Then from the angle relationship
=
XN = 12, XY = 8 + YM
=
8(8 + YM) = 192
64 + 8YM = 192
8YM = 128
YM =
= 16cm
40. Express 495g as a percentage of 16.5kg
- A. 3%
- B. 3
13 % - C. 15%
- D. 30%
Correct Answer: Option A
Explanation
The two numbers must be expressed in the same unit. To convert 495g to kg, it will be divided by 1000
495g =
= 0.495kg
To express in percentage, 0.495 will be divided by 16.5 and then multiplied by 100
% will be added to the answer
x 100
= 3%
41. Evaluate (2√3 - 4) (2√3 + 4)
- A. -4
- B. -2
- C. 2
- D. 4
Correct Answer: Option A
Explanation
2√3 - 4) ( 2√3 + 4)
= 12 + 8√3 - 8√3 – 16
= 12 – 16
= -4
The two expressions in the bracket are conjugate of each other
42. Find the equation of the tangent at the point (2, 0) to the curve y = x
- A. y = 2x - 4
- B. y = 2x + 4
- C. y = 2x - 2
- D. y = 2x + 2
Correct Answer: Option A
Explanation
The gradient to the curve is found by differentiating the curve equation with respect to x
So
The gradient of the curve is the same with that of the tangent.
At point (2, 0)
= 4 – 2 = 2
The equation of the tangent is given by (y - y1)
At point (x1, y1) = (2, 0)
y - 0 = 2(x - 2)
y = 2x - 4
43

Use the quadratic equation curve to answer this questions
What is the 80th percentile?
- A. -5.3
- B. 0.5
- C. 3
- D. 8
Correct Answer: Option A
44.
- A. -1 1
12
- B. -1
- C. 1
- D. 5
Correct Answer: Option D
Explanation
log2 8 – log3 19
= log 2 23 – log3 9−1
= log2 23 – log3 3−2
Based on law of logarithm
= 3 log2 2 – (-2 log3 3)
But log2 2 = 1,
log3 3 = 1
So, = 3 + 2
= 5
45. Tanθ is positive and Sinθ is negative. In which quadrant does θ lies
- A. Second only
- B. Third only
- C. Fourth only
- D. First and third only
Correct Answer: Option B
Explanation
First quadrant: Sin, Cos and Tan are all positive
Second quadrant: Sin is positive, Cos is negative and Tan is negative
Third quadrant: Tan is positive, Sin is negative and Cos is negative
Fourth quadrant: Cos is positive, Sin is negative and Tan is negative
The correct option is the third quadrant only where Tanθ is positive and Sinθ is negative
Correct Answer: Option B
Explanation
The total number of students is ∑ f = 3 + 4 + 5 + 6 + 7 + 6 + 5 + 4
= 40
47
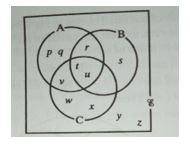
The figure below is a Venn diagram showing the elements arranged within sets A,B,C,ε.
Use the figure to answer this question
What is n(A U B)1 ?
- A. 2
- B. 3
- C. 4
- D. 7
Correct Answer: Option C
Explanation
A = (p, q, r, t, u, v)
B = (r, s, t, u)
A U B = Elements in both A and B = (p, q, r, s, t, u, v)
(A U B)1 = elements in the universal set E but not in (A U B)= (w, x, y, z)
n(A U B) 1 = number of the elements in (A U B)1 = 4
48. What is the loci of a distance 4cm from a given point P?
- A. A straight line of length 4cm
- B. a circle of radius 4cm
- C. perpendicular to point P at 4cm
- D. a circle of diameter 4cm
Correct Answer: Option B
49. Given that Sin (5
Find the value of x
- A. 14
o
- B. 21
o - C. 32
o - D. 39
o
Explanation
Sin(5x - 28) = Cos(3x - 50)………..i
But Sinα = Cos(90 - α)
So Sin(5x - 28) = Cos(90 - [5x - 28])
Sin(5x - 28) = Cos(90 - 5x + 28)
Sin(5x - 28) = Cos(118 - 5x)………ii
Combining i and ii
Cos(3x - 50) = Cos(118 - 5x)
3x - 50 = 118 - 5x
Collecting the like terms
3x + 5x = 118 + 50
8x = 168
x =
x = 21
Answer is B
50. Find the average of the first four prime numbers greater than 10
- A. 20
- B. 19
- C. 17
- D. 15
Correct Answer: Option D
Explanation
Prime numbers are numbers that has only two factors (i.e 1 and itself). They are numbers that are only divisible by 1 and their selves. First four Prime numbers greater than 10 are 11, 13, 17 and 19
Average = sum of numbers / number
=
=
= 15
51

What is the modal age?
- A. 4
- B. 5
- C. 6
- D. 7
Correct Answer: Option B
Explanation
The modal age is the age with the highest frequency, and that is age 5 years with f of 7
52. Convert 0.04945 to two significant figures
- A. 0.040
- B. 0.049
- C. 0.050
- D. 0.49
Correct Answer: Option B
Explanation
0.04945 to 2s.f is 0.049
53. Calculate 243
expressing your answer in base 10
- A. 0
- B. 1
- C. 26
- D. 46
Correct Answer: Option C
Explanation
Since they are of different base, convert to base 10
243
= (2 x 62) + (4 x 61) + (3 x 60)
= 72 + 24 + 3 = 99 base 10
243
= 2 x 52 + 4 x 51 +3 x 50
50 + 20 + 3 = 73 base 10
Subtracting them, 99 - 73
= 26
54. Evaluate ∫
- A. 1.47
- B. 2.67
- C. 3.23
- D. 3.47
Correct Answer: Option D
Explanation
∫
dx = 5 ∫
Since the integral of
is Inx
∫
dx = 5 (In<2 – InIn1)
= 3.4657
= 3.47
55. Tossing a coin and rolling a die are two separate events. What is the probability of obtaining a tail on the coin and an even number on the die?
- A.
116
- B.
16 - C.
14 - D.
38 Correct Answer: Option C
Explanation
P( tail on a coin) =
12 Even numbers on a care 2, 4 and 6
P( even number on a die) =
36 =
12 P( tail on a coin and even number on a die) =12 x
12 =14 56. Out of 7 consonants and 4 vowels, how many words of 3 consonants and 2 vowels can be formed?
- A. 210
- B. 1050
- C. 21400
- D. 25200
Correct Answer: Option A
Explanation
To form words having 3 consonants and 2 vowels out of 7 consonants and 4 vowels, the number of such words is 7/3C x 4/2C = 35 x 6
= 210
57. From a point P, R is 5km due West and 12km due South. Find the distance between P and R'.
- A. 5km
- B. 12km
- C. 13km
- D. 17km
Correct Answer: Option C
Explanation
Using Pythagoras theorem
PR
2 = 5
2 + 122 25 + 144 = 169
PR = √(169)= 13km
Answer is C
58. if y = 23
five + 101three find y leaving your answer in base two
- A. 1110
- B. 10111
- C. 11101
- D. 111100
Correct Answer: Option B
Explanation

First we convert the numbers to base ten
23
= 2 x 51 + 3 x 50
= 10 + 3 = 13
101
= (1 x 32) + (0 x 31) + (1 x 30)
= 9 + 0 + 1 = 10
So, y = 13 + 10 = 23
To convert 23 to base 2 (as in the diagram above)
Y = 23
= 10111
Answer is B
59. A box contains two red balls and four blue balls. A ball is drawn at random from the box and then replaced before a second ball is drawn. Find the probability of drawing two red balls.
- A.
23
- B.
13 - C.
14 - D.
19
Correct Answer: Option D
Explanation
Total number of balls = 2 + 4 = 6
P(of picking a red ball) =
=
=
P( picking two red balls) =
×
60

Which one of the following gives the members of the set A1 n B n C?
- A. Φ
- B. {s}
- C. {t, u}
- D. {y, z}
Correct Answer: Option A
Explanation
A1 = Elements in the universal set but not in A = {s, w, x, y, z}
B = {r, s. t, u}
C = {t, u, v, w, x}
A1 n B n C = elements common to the three sets = none = empty set = Φ
61. If P = [
make T the subject of the relation
- A. T =
R+P315Q - B. T =
R−15P3Q - C. T =
R−15P3Q - D. T =
15R−QP3
Correct Answer: Option C
Explanation
Taking the cube of both sides of the equation give
P
=
15P
= Q(R - T)
Divide both sides by Q
= R - T
Rearranging gives
T = R -
=
Answer is C
62. Calculate the area of an equilateral triangle of side 8cm
- A. 8√3
- B. 16
- C. 4√3
- D. 16√3
Correct Answer: Option D
Explanation
An equilateral triangle has all sides equal and all angles equal as 600
Area =
absinθ
Area =
x 8 x 8 x sin60
=
x 64 x
63. The pie chart shows the monthly expenditure of a public servant. The monthly expenditure on housing is twice that of school fees. How much does the worker spend on housing if his monthly income is ₦7200?
- A. ₦1000
- B. ₦2000
- C. ₦3000
- D. ₦4000
Correct Answer: Option B
Explanation
Let the monthly expenditure angle for school fees is x, then that of housing will be 2x. Since the total angle in the circle is 360.
Using 90 as the angle for transport
So, x + 2x + 120 + 90 = 360
3x + 210 = 3605
3x = 360 - 210
= 150
x =
= 50
So the angle for housing is 2x = 2 × 50
= 100
Amount spent on housing =
× 7200
= ₦2000
64.Approximate 0.9875 to 1 decimal place.
- A. 1.1
- B. 1.0
- C. 0.9
- D. 0.10
Correct Answer: Option B
Explanation
9 is on one decimal place, the next number to it is 8 which will be rounded up to 1 because it is greater than 5 and then added to 9 to give 10, 10 cannot be written, it will then be rounded up to 1 and added to 0.
So the answer is 1.0
65.Simplify 25
- A. 1
14 - B. 2
14 - C. 6
- D. 10
Correct Answer: Option A
Explanation
Using law of indices
25
× 8
) -2
= 5 x 2-2
= 5 x
=
=
66. From a point R, 300m north of P, a man walks eastwards to a place; Q which is 600m from P. Find the bearing of P from Q correct to the nearest degree
- A. 026
o
- B. 045
o - C. 210
o - D. 240
o
Correct Answer: Option D
Explanation
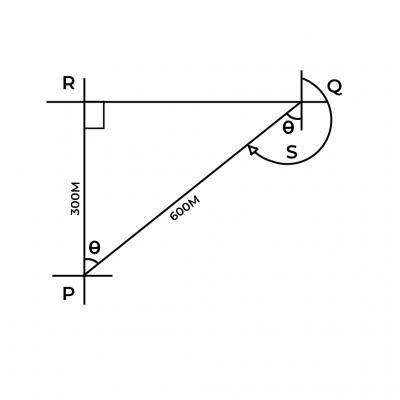
Cos θ =
=
= 0.5
θ = Cos - 10.5
= 60
∠ RPQ = ∠ PQs
So the bearing of P from Q is 180 + 60 = 240
Answer is D
67. Add 54
giving your answers in base eight
- A. 111
- B. 121
- C. 123
- D. 143
Correct Answer: Option D
Explanation
54
and 67
Starting with normal addition, 4 + 7 gives 11
(it is more than the base, 8) 8 goes in 11 just 1 time, remaining 3, the remainder will be written, and the 1 will be added to the sum of 5 and 6 which gives 12 altogether, 8 goes in 12 one time remaining 4, the remainder 4 was written and then the 1 that was the quotient was then written since nothing to add the 1 to.
So answer is 143 in base eight.
Related Posts
Subscribe Our Newsletter

0 Comments to "Mathematics JAMB CBT Practice Questions & Answers 2021 SET 1"
Post a Comment